Probability(x): Where is the oscillating particle?

For the oscillator in the $n^{th}$ eigenstate with energy $E_n$, the probability of being between x and $x + dx$ is $\Psi_n^* \Psi_n dx$. In the following figure, $\Psi_n^* \Psi_n$ is plotted in solid lines for $n = 0$ (red) and for $n = 5$ (blue), along with the corresponding classical predictions, plotted in dotted lines for classical oscillators with the same total energies (0.5 hf and 5.5 hf). Numerical constants chosen: m = mass of H atom, f = 100/ps, about the frequency of O-H bond stretching.

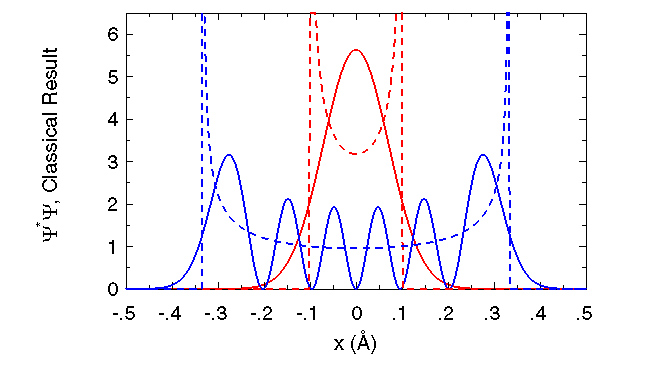
Quantum and classical probability of finding oscillator as a function of position.

Classically, the probability that the oscillating particle is at a given value of x is simply the fraction of time that it spends there, which is inversely proportional to its velocity $v(x)$ at that position. The particle must stop completely (for a moment) before reversing its direction, and so it spends the most time where the spring is either fully compressed or fully extended ($x = \pm A$). It spends the least time where its velocity is greatest, i.e., where the spring is at its equilibrium length ($x = 0$). Classically, there is zero chance for a particle to have a potential energy V greater than its total energy E, and so the motion is strictly confined to the range $-A \le x \le A$ (see vertical dotted lines at $x = \pm A$).

Quantum mechanically, there exist states (any n > 0) for which there are locations x, where the probability of finding the particle is zero, and that these locations separate regions of high probability! Also, note that there is appreciable probability that the particle can be found outside the range $-A \le x \le A$, where classically it is strictly forbidden! This quantum mechanical tunneling is related to the famous Heisenberg Uncertainty Principle, which states that one cannot know both the position and momentum of a particle with infinite precision at the same time.

Finally, note that the classical approximation more closely resembles the average of $\Psi_n^* \Psi_n$ as the energy $E_n$ of the oscillator increases.