Average Energy U and Heat Capacity

Next, we consider the average energy $U = \langle E \rangle$ of the harmonic oscillator at fixed $N\cal{V}\it {T}$. We calculate it as a function of temperature using equations 2, 4, and 5, with the observable $O = E$. U is plotted in dotted lines for three frequencies (spring constants k): f = 100/ps (green), 10/ps (blue), and 1/ps (cyan). The classical result (red) is $U = k_B T$, regardless of the frequency of the harmonic motion (the classical `equipartition of energy'). Note that the deviation of classical from quantum behavior is reduced as the frequency, and hence the energy gap between quantum states, is reduced.

Harmonic oscillation: average energy and heat capacity versus temperature

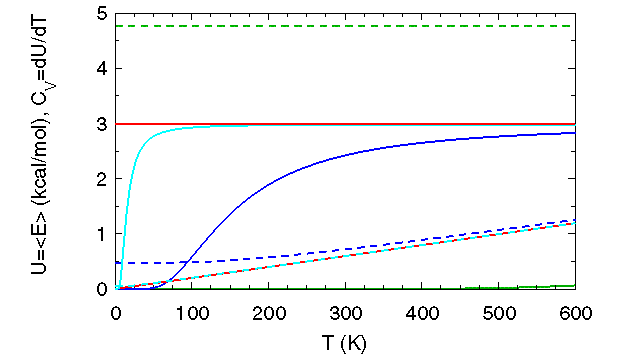
The heat capacity at constant volume, $C_V = \partial U/\partial T\vert _V$, is plotted in solid lines for each of the four U curves. (To fit on the same scale, $C_V$ values were scaled by a factor of 1500). This heat capacity measures the energy absorbed (released) by a system as its temperature is raised (lowered) one degree while the volume is held constant. (The heat capacity at constant pressure, $C_P$, is larger than $C_V$ for gases because work is done as the container expands.) In the early twentieth century, experimentally measured values of the heat capacity for solids at low temperature, which can be approximated as three dimensional arrays of harmonic oscillators, pointed to a problem in the classical theory. Classical physics predicted a temperature independent heat capacity (red horizontal line), whereas the measured values went to zero as $T^3$ as $T \rightarrow 0$. The much improved predictions (by Einstein in 1907 and Debye in 1912) of the low-temperature heat capacity of solids were among the earliest successes for theoretical models that invoked quantum concepts.

Harmonic oscillation at 300 K: average energy and heat capacity versus frequency

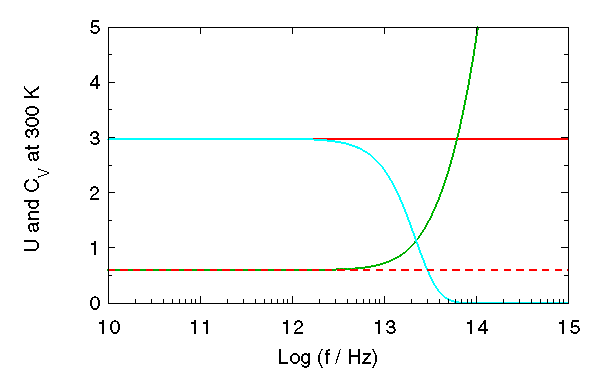
The frequency dependencies of U and $C_V$ at 300 K are shown in the second figure. Again, the $C_V$ values have been scaled by a factor of 1500. Classical quantities (in red) are independent of frequency, with $U = k_B T$ = 0.6 kcal/mol drawn as a dashed line. Quantum mechanical quantities (U in green, $C_V$ in cyan) deviate more and more from classical values as the frequency is increased. As foreshadowed in the introduction, this deviation becomes appreciable at frequencies above 1/ps.

An aside: For those unfamiliar with the concept of heat capacity, consider the well known consequences of water's large heat capacity. Water can absorb and release considerable thermal energy with little change in temperature. Hence, average coastal temperatures are cooler in summer than inland temperatures as the water absorbs and stores heat. The reverse is true in winter, as the water releases the stored energy.